
ESS124
Prof. Jin-Yi Yu
Coriolis Force (for n-s motion)
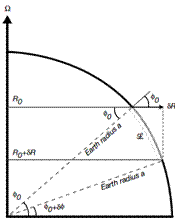
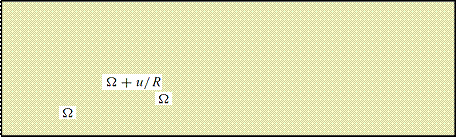
Suppose that an object of unit mass, initially at
latitude φ moving zonally at speed u, relative to the surface of the earth, is displaced in latitude or
in altitude by an impulsive force. As the object is displaced it will conserve its angular
momentum in the absence of a torque in the east–west direction. Because the distance R to the axis
of rotation changes for a displacement
in latitude or altitude, the absolute angular velocity ( ) must change if the object is to conserve its absolute angular
momentum. Here is the angular speed of rotation of the earth. Because is constant, the relative zonal
velocity must change. Thus, the object behaves as though a zonally directed
deflection force were acting on
it.
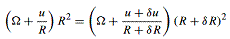

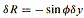
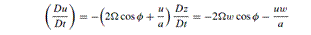


using
è
è

(neglecting
higher-orders)