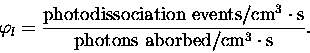Photodissociation

A general photolysis reaction is written as,

$$\rm AB + h\nu \longrightarrow \rm A + B$$ (3.18)

which is the combination of two steps: the first is the absorption of a photon, equation (2.17). The second step, shown in Table 2.1, is an electronic transition to an unbound excited state. Kinetic energy may be imparted to the photolysis fragments and/or they may be in an excited vibrational or electronic state. Note that in some cases it is possible for photodissociation events to occur upon photon fluorescence, an example of which is H₂ in Jupiter's atmosphere.

In general, the energy requirement is such that the absorption must correspond to the excitation between electronic energy levels. Minimum energies are 1 eV (election volt) or 2 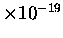$\times 10^{-19}$ J, which correspond to a wavelength of about 1 $\mu$m.

Photodissociation reactions are treated as elementary, or single-body, reactions and their rate of chemical change can be quantified by considering the rate of change in concentration of species l,

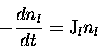$$-\frac{dn_l}{dt}= {\rm J}_l n_l$$ (3.19)

where ${\rm J}_l$ represents the photolysis rate constant (or J-value) and has units of s^-1. Equation (2.19) admits the solution 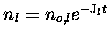$n_l=n_{o,l} e^{-{\rm J}_lt}$. Thus, J_l^-1 can be interpreted as the lifetime of species l against photolysis. One major application of solar radiative transfer is in the calculation of photolysis rate coefficients.

For a given wavelength interval, the J-value for species l is proportional to the mean radiance, the absorption cross-section, and the quantum efficiency. Considering the entire spectrum, the total J-value is given by,

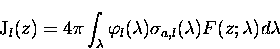$${\rm J}_l(z) = 4\pi \int_{\lambda} \varphi_l(\lambda) \sigma_{a,l}(\lambda) F(z;\lambda) d\lambda$$ (3.20)

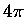where $\varphi_l(\lambda)$ is the photolysis quantum yield for species l. The factor of $4\pi$ arises from the definition of the mean radiance. Equation (2.20) represents the probability that the absorption of a single photon will lead to a photodissociation event. It is defined mathematically as,

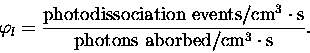$$\varphi_l = \frac{ {\rm photodissociation \, \, events/cm^3 \cdot s} } { {\rm photons \, \, aborbed/cm^3 \cdot s} }.$$ (3.21)

Quantum yield is a molecular property which can be measured through laboratory experiments and, in general, depends on pressure, temperature, and wavelength. Note that J_l is independent of the concentration of species l except when absorption is significant enough to affect the mean radiance or if the cross-section or quantum yield are pressure dependent (e.g.: O₂ at high pressure).